At high intensity, laser light substantially modifies the optical properties of material it passes through. The refractive index of the material has a term proportional to the laser intensity, and hence variations in the laser spatial and temporal profile lead to focusing and defocusing of pulse energy. A number of effects result from this phenomenon, including self-focusing of laser light, or modulation of the laser’s temporal envelope.
Laser Filament Production
The desire to probe phenomena on ever shorter timescales has encouraged the development of a number of techniques for generating ultrashort laser pulses. One of these techniques, filamentation compression, has been implemented by researchers at CUOS on the λ3 laser system to reduce pulse durations from 30 fs to less than 10 fs.
Filamentation of a high power laser pulse occurs when a balance is struck between two competing nonlinear processes: self-focusing (caused by a third-order atomic non linearity present in all materials) and plasma defocusing (caused by the difference in refractive index of a plasma due to the density of electrons). When self-focusing and plasma defocusing are balanced a pulse can travel exceptionally long distances (kilometers in some cases) without expanding.
Filamentation compression occurs when a filamenting pulse simultaneously experiences temporal nonlinearities. Self phase modulation (SPM), self steepening, and ionization blue-shifting are examples of nonlinearities that occur during filamentation which increase the bandwidth of the pulse and allow it to be compressed to a shorter duration. Further manipulation of the pulse following filamentation is often required to fully utilize the increased bandwidth, but under the right conditions a pulse can undergo self-compression during filamentation leading to a sub-10 fs pulse with no extra manipulation.
Taken to its limit, filamentation compression may be able to produce high-power (> 1 TW) pulses with single-cycle durations (i.e. a pulse consisting of a single oscillation of the electric field). Compared to many-cycle pulses, single-cycle pulses can lead to significantly different laser-plasma interactions in experiments on, for example, high-order harmonic generation and charged particle acceleration.
Non‐linear Optics in Plasma
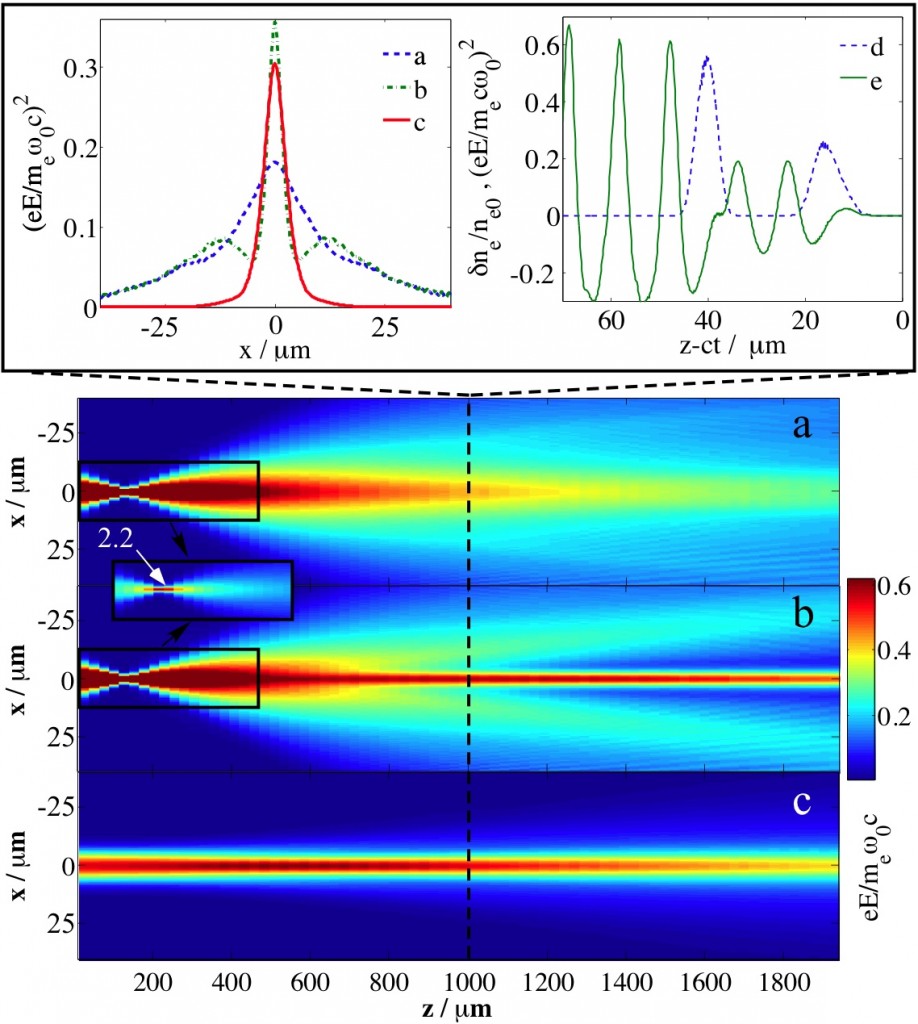
Underdense plasma acts as a non-linear medium for the propagation of electromagnetic radiation. The dispersion relation for electromagnetic waves propagating in plasma is given by ω2 = k2c2 + ωp2,where ωp = n0e2meε0 is the frequency of a plasma wave with local electron number density n0. Due to the mass difference, the ions generally have little influence on the dispersion of electromagnetic waves. If ωp exceeds ω, the wave can no longer propagate and an evanescent wave results. If the oscillation of the electrons is relativistic, then the inertial mass of the electrons will increase, which affects the plasma frequency. This in turn affects the local phase velocity (and hence refractive index) of the plasma, as the phase velocity is given by ω/k. In addition to the relativistic mass increase of electrons oscillating in the laser field, density perturbations also result in a spatially varying refractive index. At very high intensities, the ponderomotive force, associated with the energy density gradients in the pulse, acts to expel charged particles from regions of high intensity. This creates significant density gradients, and hence refractive index gradients, which in general proceed to focus the radiation. Hence, the medium then acts as a positive lens for both relativistic mass increase and ponderomotive cavity formation. It may be shown that when the laser power exceeds a threshold critical value Pcr ≈ 17(ω/ωp)2 GW, the laser pulse experiences relativistic self-focusing, and can self-channel through the plasma.
Laser pulse guiding is an important and useful feature of the non-linear optical effects of plasma. Optical guiding of high intensity pulses has been experimentally demonstrated in preformed channels [Butler PRL 2002], by the self-guiding of a pulse in a laser wakefield accelerator [Thomas PRL 2007a], and by plasma wave-guiding of a pulse by the wakefield of a second pulse [Thomas PRL 2008]. Guiding of a pulse in a plasma is already a key feature of laser wake-field acceleration, where it is used to extend the propagation length, and hence acceleration length. Greater than GeVenergy electron beams have been demonstrated by guided laser wakefield acceleration [Leemans NP 2006].
A number of theoretical papers have predicted interesting optical effects in underdense plasmas, in addition to optical guiding, due to the high energy density of the laser and the non-linear plasma medium. These include, self-compression due to photon acceleration [WilksPRL 1989], mutual attraction of laser beams [Ren POP 2002], the use of plasma as a lens [Ren PRE 2001a], multi-filamentation [Cattani PRE 2001], self-channeling and plasma wave guiding [Sprangle PRL 1992, Electron cavity, Lu POP 2006], laser hosing [Duda PRL 1999] and laser amplification [Malkin PRL 1999], to name but a few.
